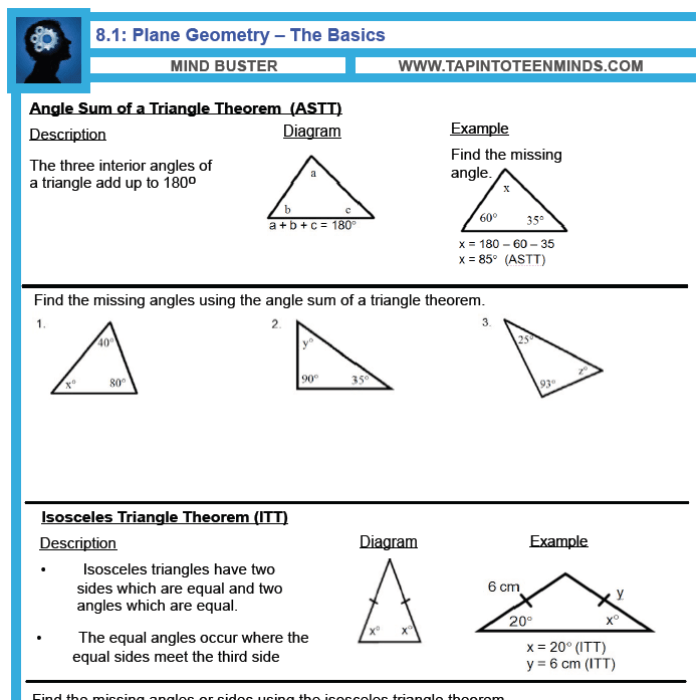
Topic 3 line and angle relationships – Line and angle relationships form the cornerstone of geometry, shaping our understanding of spatial arrangements and providing a framework for analyzing the world around us. This intricate interplay governs the behavior of lines and angles, revealing fundamental properties and applications that extend beyond the classroom.
From parallel lines that never meet to perpendicular lines that intersect at right angles, the relationships between lines and angles paint a captivating tapestry of geometric patterns. Intersecting lines create angles that can be classified as complementary, supplementary, or vertical, each with unique characteristics and applications.
3 Line and Angle Relationships: Topic 3 Line And Angle Relationships
Line and angle relationships are fundamental concepts in geometry that describe the geometric arrangement and relationships between lines and angles. These relationships form the basis for understanding the properties of geometric figures, solving geometric problems, and making predictions.
1. Definition of Lines and Angles
A line is a one-dimensional geometric object that extends infinitely in both directions. It is defined by two distinct points and has no thickness or width. An angle is formed by two rays that share a common endpoint, called the vertex.
The measure of an angle is determined by the amount of rotation between the two rays, and it is expressed in degrees or radians. Angles can be classified as acute (less than 90 degrees), obtuse (greater than 90 degrees), or right (exactly 90 degrees).
2. Types of Line and Angle Relationships, Topic 3 line and angle relationships
Parallel lines are lines that lie in the same plane and never intersect. Perpendicular lines are lines that intersect at a right angle. Intersecting lines are lines that intersect at a point that is not a right angle.
3. Properties of Angle Relationships
Complementary angles are two angles whose sum is 90 degrees. Supplementary angles are two angles whose sum is 180 degrees. Vertical angles are two angles that are formed by two intersecting lines and are opposite each other. Vertical angles are always congruent, meaning they have the same measure.
4. Applications of Line and Angle Relationships
Line and angle relationships are used in various real-world applications, including geometry, architecture, and engineering. In geometry, they are used to solve geometric problems, such as finding the area of a triangle or the volume of a cone. In architecture, they are used to design buildings and structures that are stable and aesthetically pleasing.
In engineering, they are used to design bridges, roads, and other structures that are safe and efficient.
Quick FAQs
What are parallel lines?
Parallel lines are two lines that never intersect, no matter how far they are extended.
What is the difference between complementary and supplementary angles?
Complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees.
What are the properties of vertical angles?
Vertical angles are opposite angles formed by two intersecting lines, and they are always congruent.